What is Arc Length?
Arc length is the distance between two points along a section of a curve.
Or
Arc length can also be defined as the distance along the part of the circumference of a circle.
Arc Length Formula
Arc length can be calculated with the help of the central angle of that arc and the radius of the circle to which that arc belongs. The unit of the central angle can be in degrees or radians. So, depending upon the unit of the central angle the length of the corresponding arc can be found out using different formulas as mentioned below:
When the unit of central angle is in radians then
Arc length, s = θ × r
When the unit of central angle is in degrees then
Arc length, s = 2πr (θ/360o) = πr (θ/180o)
Where,
s = Length of arc
θ = Central Arc Length of Arc
r = Radius of the circle
Arc length of a curve in integral form:
The arc length of a curve is given as
Where,
f′(x) = First integral of the given curve whose arc length is to be calculated
b = It is the upper limit of our definite integral that is the rightmost point of the given arc
a = It is the lower limit of our definite integral that is the leftmost point of the given arc
1. Let the angle subtended by an arc at the centre of the circle be 120o. If the circumference of the circle is 54 cm then find the arc length.
Solutions:
Given,
Central angle, θ = 120o
Circumference of circle, 2πr = 54 cm
Thus, arc length is
Thus the arc length comes to be 18 cm.
2. Find the central angle subtended by an arc whose length is 1/4 of the circumference of the corresponding circle.
Solution:
We know that circumference of the circle, C = 2πr
And Arc length, s = 2πr (θ/360o)
According to the question,
Hence, the central angle = 90o.
3.Find the arc length of the curve y = 4 between points x = 0 and x = 4.
Solution:
Given, f(x) = 4
Therefore, the required arc length is 4 units.
To get more such useful articles and important resources for CBSE Board Exam or Competitive Exam preparations, visit jagranjosh.com.

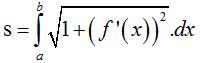



Comments
All Comments (0)
Join the conversation