CTET 2021 Exam Memory Based Mathematics Questions with Answer Keys PDF Download: Central Teacher Eligibility Test (CTET) 2021 Exam is being conducted by CBSE in online mode till 13th January 2022 for Primary (Paper-1) & Upper Primary Levels. To crack the CTET 2021 Exam, candidates must practice the memory-based questions of different sections of the exam. It will help them in improving their speed of attempting maximum questions in minimum time with accuracy.
| Recent Stories |
| Check CTET 2021 Memory Based PEDAGOGY Questions with Answers |
Let’s have a look at the Important Memory Based Questions that are being covered in the CTET 2021 Exam.
CTET 2021 Exam Memory Based Mathematics Questions with Answers |
1. The numbers of boys and girls in a college are in the ratio of 3:2. If 20% of the boys and 25% of the girls are adults, the percentage of students, who are not adults, is
- 58%
- 60(1/5)%
- 78%
- 83(1/3)%
Answer: (c)
Explanation: Let the number of total boys and girls is 100
Boys : Girls = 3 : 2 -> Sum of ratio terms = 3 + 2 = 5
Numbers of boys = 100 x (3/5)= 60
and number of girls = 100 x (2/5) = 40
The number of adults = (60 x 20)/100 + (40 x 25)/100 = 12 + 10 = 22
The number of non-adults = 100-22=78
Percentage of student, who are not adults = 78 %
2. P, Q, R are employed to do a work for Rs. 5750. P and Q together finished 19/23 of work and Q and R together finished 8/23 of work. Wage of Q, in rupees, is-
- 2850
- 3750
- 2750
- 1000
Answer: (d)
Explanation: Work done by Q = 19/23 + 8/23 – 1 = 4/23
We know Wage of Q = 4/23 × 5750
= Rs. 1000
3. A boy and a girl together fill a cistern with water. The boy pours 4 litres of water every 3 minutes and the girl pours 3 litres every 4 minutes. How much time will it take to fill 100 litres of water in the cistern?
- 36 minutes
- 42 minutes
- 48 minutes
- 44 minutes
Answer: (c)
Explanation: Water filled by 1 boys in 1 min = 4/3 litres and water filled 1 girl 1 min = ¾ litres. In 1 min. Water filled by both = 25/12 litres. Time taken to fill 100 litres = 100 x (12/25) = 48 min
4. A jar contains a mixture of 2 liquids A and B in the ratio 4:1. When 10litres of the mixture is taken out and 10 litres of liquid B is poured into the jar, the ratio becomes 2:3. How many litres of the liquid A was contained in the jar?
- 17 litres
- 16 litres
- 18 litres
- 15 litres
Answer: (b)
Explanation: In original mixture, % of liquid B = 1/(4 + 1) × 100 = 20%
In the resultant mixture, % of liquid B = 3/(2 + 3) × 100 = 60%
Replacement is made by the liquid B, so the % of B in second mixture= 100%
Then by the method of allegation:
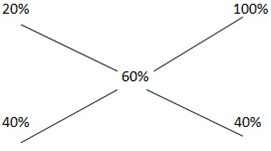
Ratio in which first and second mixtures should be added is 1:1. It implies that the reduced quantity of the first mixture and the second mixture and the quantity of mixture B which is to be added are the same.
Total mixture = 10+10 = 20 litres.
and liquid A =(20/5) 4 = 16 litres
| CTET Study Material |
| CTET 2021 Important Child Development and Pedagogy Preparation Tips |
Directions (5 – 7): What should come in place of the question mark (?) in the following number series?
5. 4, 24, 120, 480, 1440, 2880, ?
- 3600
- 4800
- 2880
- 5760
Answer: (c)
Explanation: The terms are multiplied by 6, 5, 4, 3 and so on.
6. 11, 23, 48, 99, 202, 409, ?
- 818
- 820
- 824
- 832
Answer: (C)
Explanation: 23 = 11 × 2 + 1; 48 = 23 × 2 + 2; 99 = 48 × 2 + 3; 202 = 99 × 2 + 4; and so on.
7. 11, 17, 23, 31, 41, 47, ?
- 51
- 59
- 61
- 67
Answer: (b)
Explanation: All numbers are prime numbers with one prime number missing between any pair of consecutive terms.
8. In an examination, 34% of the students failed in mathematics and 42% failed in English. If 20% of the students failed in both the subjects, then find the percentage of students who passed in both the subjects.
- 40%
- 41%
- 43%
- 44%
Answer: (d)
Explanation: Failed in mathematics, n (1) = 34
Failed in English, n(2) = 42
n(AUB) = n(1) + n (2) – n (A∩ B )
= 34 + 42- 20 = 56
Failed in either or both subjects are 56
Percentage passed = (100−56)%=44%
9. Two train of equal lengths are running on parallel lines in the same directions at the rate of 92 km/h and 72 km/h respectively. The faster train passes the slower train in 54 seconds. The length of each train is
- 500 m.
- 180 m.
- 150 m.
- 240 m.
Answer: (C)
Explanation: Speed of faster train relative to slower train
= (92 – 72) km/h = 20 km/h
= 20 ×(5/18) = 50/9 m/s
Distance covered in 54 seconds = (50/9) X 54 m = 300 m
2(length of each train) = 300m
length of each train = 150 m
10. 4 men can complete a piece of work in 2 days. 4 women can complete the same piece of work in 4 days whereas 5 children can complete the same piece of work in 4 days. If 2 men, 4 women and 10 children work together, in how many days can the work be completed?
- 1 day
- 3 days
- 2 days
- 4 days
Answer: (a)
Explanation: 4 M × 2 = 4 W × 4 = 5 C × 4
2 M = 4 W = 5 C
4 W × 4 = 16 W × 1 = 1 day
11. If a : 5 = b : 7 = c : 8, then (a + b + c)/a is equal to
- 2
- 4
- 7
- 1/4
Answer: (b)
Explanation: Given, a : 5 = b : 7 = c : 8
Let a/5 = b/7 = c/8 = k
Then, a = 5k, b = 7k, c = 8k
Now, (a + b + c)/a = k(5 + 7 + 8)/5k = 4
12. A man rows at 5 km/h in still water. If the river is running at 1 km/h, it takes him 75 minutes to row to a place and back. How for is the place?
- 5 km
- 3 km
- 4 km
- 5 km
Answer: (b)
Explanation: X/(5+1) + X/(5-1) = 75/60
or, X/6 + X/4 = 5/4
4x + 6 x / 24 = 5/4
10x = 30
x = 3 km
13. 7,500 is borrowed at C.I. at the rate of 2% for the first year, 4% for the second year and 5% for the third year. The amount to the paid after 3 years will be
- 8235.00
- 8432.00
- 8520.20
- 8353.80
Answer: (d)
Explanation: Amount = P (1 + r1/100) (1 + r2/100) (1 + r3/100)
= Rs. 8353.80
14. Imran borrowed a sum of money from Jayant at the rate of 8% per annum simple interest for the first four years, 10% per annum for the next six years and 12% per annum for the period beyond 10 years. If he pays a total of Rs. 12,160 as interest only at the end of 15 years, how much way did he borrow?
- 8000
- 10,000
- 12,000
- 9,000
Answer: (a)
Explanation: Let the principal = Rs. P
(P x 8 x 4)/100 + (P x 10 x 6)/100 + (P x 12 x 5)/100 = 12160
152P = 12160 × 100
P = (12160 X 100) / ( 152) = Rs. 8000
15. The question below consists of a question and two statements numbered I and II given below it. You have to decide whether the data provided in the statements are sufficient to Answer the questions. Give Answer –
- If the data in statement I alone are sufficient to Answer the question, while the data in statement II alone are not sufficient to Answer the question.
- If the data in statement II alone are sufficient to Answer the question, while the data in statement I alone are not sufficient to Answer the question.
- If the data either in statement I alone or in statement II alone are sufficient to Answer the question.
- If the data in both the statements I and II together are necessary to Answer the question.
Question: Which of the integers a, b, c, d, and e are odd numbers?
- a, b, c, d, and e are consecutive integers.
- C is an even integer.
Answer: (d)
Explanation: With the help of statement I and II using together, odd number out of a, b, c, d and e can be found out.
Comments
All Comments (0)
Join the conversation